1. What is continuity? What is discontinuity?
A continuous function is predictable. That means there are no breaks, holes, and jumps. The function can be drawn without lifting the pencil. Here's an example of a continuous function.
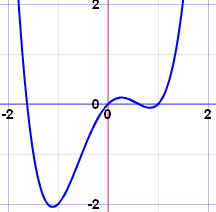 |
| http://www.mathsisfun.com/calculus/continuity.html |
On the other hand, a discontinuous function is unpredictable and may have breaks, holes or jumps. There are two families of discontinuities, removable and non-removable. A point discontinuity is a removable discontinuity known as a hole. For non-removable, there are jump discontinuities, oscillating behavior and infinite discontinuities known as unbounded behavior (it has a vertical asymptote).
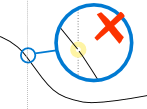 | ||||
|
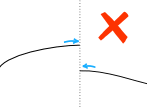 | |
|
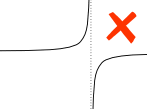 | |
|
2. What is a limit? When does a limit exist? When does a limit not exist? What is the difference between a limit and a value?
A limit is the height a function intends to reach (y-value). It is read as "The limit as x approaches 'a number' of f(x) is equal to 'L' ". A limit exists as long as you reach the same height from both the left and the right. This means both the left and right limit must be the same. If the graph does not break at a given x-value, then the limit will exist there. This means that a limit will exists in functions with holes. However, a limit will not be reached in non-removable discontinuities such as jumps, oscillating behavior, or infinite discontinuities. For infinite discontinuities, we say the limit is unbounded (or it does not exist) because infinity is not a number. This is because they do not reach the same height from both the left and right side. However, we can still write one-sided limits.While limit is the intended height, the value is the actual height. This means that the limit and value can be the same or different depending on the function. Sometimes, there may be no actual value, as in the case with 2 open circles.
 |
| http://www3.ges.sd23.bc.ca/classes/calculus/unit2/U02L02.html |
In the image above, you can see the limit at a is L. However L is not the actual value, it is just the intended height. Instead the value is b, in which there is a closed circle.
3. How do we evaluate limits numerically, graphically, and algebraically?
To evaluate limits numerically, you will have to begin by drawing a table with 3 left and 3 right of number x is approaching. The values on the ends should be a tenth away from the center, and the closer you get to the center, the closer the x-value should be. You would then plug the function into your graphing calculator and trace the location of the x-value. An example can be seen below. However, you should note that the limit as x approaches from the left is 12 while from the right is 4.
|
|
http://people.hofstra.edu/stefan_waner/realworld/tutorials/frames2_6a.html
To evaluate limits graphically, plug the function into the y= screen on the graphing calculator. Then you can either go to tblset and make the ind. variable ask and then type in the values close to the limit or you can hit trace. To find the limit, put your finger on a spot to the left and to the right of where you want to evaluate the limit and then move them together to find where they meet. If they don't, then there is no limit.
https://www.youtube.com/watch?v=UkjgJQaGx98
Notice in the youtube video above how Patrick in the middle moves his fingers closer and closer to the x-value he wants to approach from both sides.
When solving for limits algebraically, you need to begin with the method of substitution. Basically, this is taking the number the limit is approaching and plugging it in anywhere you see x. Through substitution, we can get 4 types of answers:
1. a numerical answer- we're done
2. 0/# -this is 0 -we're done
3. #/0 which is undefined and the limit does not exist-we're done
4. 0/0 indeterminate form - use another method
The image below provides a function in which substitution is used to find the limit.
 |
| http://www.onlinemathlearning.com/limits-calculus.html |
 |
| http://www.drcruzan.com/MathLimits.html |
If you are unable to factor the function, they you will have to use the rationalizing/conjugate method. First you multiply the top and bottom by the conjugate . Then you simplify by foiling the value that was used to find the conjugate while leaving the other factored. Then there should be something you can cancel so that you can use substitution to find the value.The image below provides an example of multiplying the numerator and denominator by the conjugate, rad(x^2+9)=3 .
| http://www.onlinemathlearning.com/limits-calculus.html |
References:
http://www.mathsisfun.com/calculus/continuity.html
http://www3.ges.sd23.bc.ca/classes/calculus/unit2/U02L02.html
http://people.hofstra.edu/stefan_waner/realworld/tutorials/frames2_6a.html
https://www.youtube.com/watch?v=UkjgJQaGx98
http://www.drcruzan.com/MathLimits.html
http://www.onlinemathlearning.com/limits-calculus.html